26 Jun 2019
Interview
NORA OLEARIUS: THE LOGICALLY JUSTIFIABLE AND THE SENSUALLY PERCEPTIBLE
Visual artist Nora Olearius is a HIAP resident for three months from the beginning of June until the end of August. She is staying at HIAP’s Cable Factory studios. During her stay in Helsinki, she is exploring fractal geometry and their connections to Finnish nature, connections between mathematics and art, and the Yoik, the traditional singing of the Sámi people.

Frappé, wallpapered notes, engraving, 365cm x 421cm x 80cm, 2017
AA: What are you working on while at HIAP?
NO: While I am at HIAP I will focus on fractal geometry as well as the Yoik, the traditional singing form of the Sámi people. I believe there are several points of contact between natural fractal patterns and the structure of the Yoik and my concern is to find a way of visualizing them. Therefore, I hope that I will have the opportunity to better understand the Yoik and its cultural function. This is the content framework, but what will happen within this framework has yet to evolve.
AA: You are interested in numerical representations and mathematical calculations. What about mathematics attracts you?
NO: It is mostly not mathematics alone that attracts me, but the transfer of mathematical concepts and rules into areas where it may seem strange.
I am mainly interested in investigating the field of tension between the rationally justifiable and the sensually perceptible. Besides, mathematics constantly confronts me with new riddles; it can be helpful in solving riddles, but at the same time it is one of them. I like riddles.

Frappé, detail
AA: Mathematics is about abstract reasoning, and that can be said about art, too. More broadly, how do you see maths’ role in our lives?
NO: Mathematics plays a key role in the world, almost everything can be considered mathematically. We’re constantly surrounded by numbers. The language of mathematics is applicable in a variety of fields, it only depends on translation and creating connections, even if they sometimes seem strange. Furthermore, it is a powerful tool to try to explain and understand the system in which we live. But maybe mathematic can only explain so much because it was thought up exactly for this purpose…the numbers and formulas have been optimized for exactly these questions.
When it comes to abstract reasoning, I believe that the term means something different for art than for mathematics. Considering the origin of the word from the Latin it seems to be a matter of deducting or removing something. If abstract thinking means to reduce something to the essential and to convert it into something more general, I find it easier to transfer this thought process into mathematics. Abstraction in art, I believe, always requires the ability to interpret, it is then more a matter of adding than of omitting.
Or is it rather about idealized thought constructions? You have caused quite a mess in my head with this question :-). I will continue to think about it…
AA: How do you feel fractal patterns compare with other aesthetic/mathematical concepts, for example the golden ratio and symmetry?
NO: I think that they are closely connected and that it is difficult to think one without the other. On the emotional level, they create a harmonious and aesthetic sensibility. The golden ratio consists of asymmetric parts, but their relation to each other is symmetric. The small part relates to the large part, as the large part relates to the whole part. This direct connection between the major and the minor can also be understood as fractal connectivity. Furthermore, there are several fractals that are scaled according to the golden ratio, such as the golden tree.

Cubicle x Factor, rented paintings, cardboard boxes –›cubicle floor area 8m2, side height 150cm fineliner on paper, 9 sheets 21cm x 15cm each, oil on canvas 50cm x 50cm, 2018, photo: Franziska von den Driesch
AA: Have you studied mathematics? When did you first realise you were interested in working in-between the two fields of maths and art? Where did you get all the knowledge about physics and mathematics required in your research?
NO: My interest in the work between mathematics and art has slowly grown, but I think it arose from the question of the justifiably of my artistic decisions. I began to observe my environment and my decisions through “numerical glasses”, and very soon I came up against the boundary of considering art on the basis of pure logic, and it is precisely this tightrope walk that interests me.
The mathematical and physical knowledge I need for my research is at a basic level, I’d say. There is still a lot of room for improvement! To increase my knowledge, I started studying physics in 2018, but I have to admit, I wasn’t a very outstanding student. But while I was sitting in the lectures a lot happened in my head, and that’s something good. But most of the information I need I find in books, mostly in the never-ending book of the Internet.

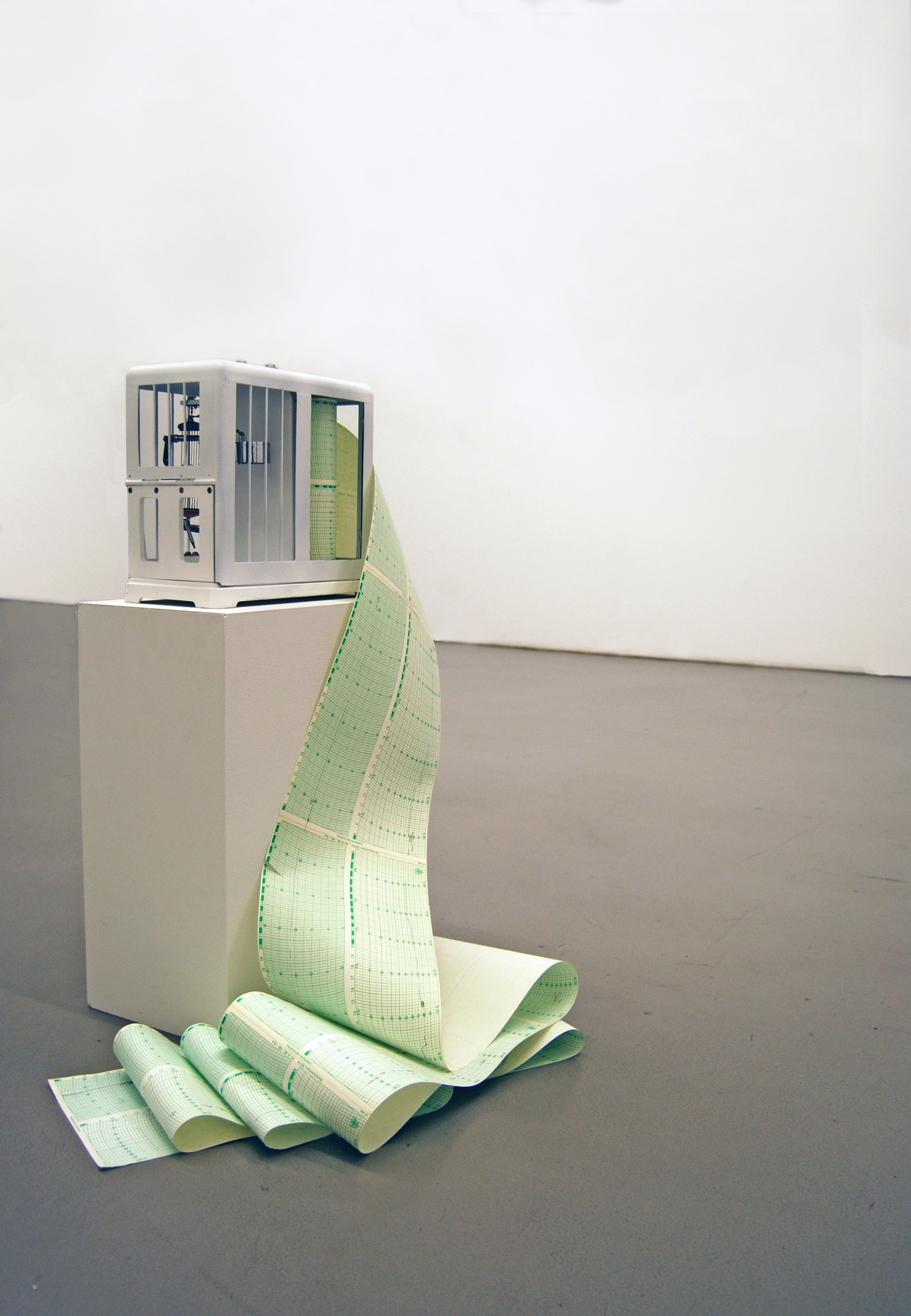
Hairmatic, thermo-hygrograph, artist‘s hair, oil on canvas,fineliner on paper, 2017, photo Christina Stohn
AA: Tell us a bit about the work you produced during 2018 at the Bremen Studio Stipend. You worked with previous HIAP resident Nadja Quante, isn’t that right?
NO: Yes, thats right! Nadja and I worked quite closely together during my Studio Stipend, door to door so to speak. During this time I have been concerned with the question of how the monetary value of art can be calculated and presented. The outcome of this consideration is a work with the title Cubicle x Factor. Nadja has written a text about it where the wording is on point and therefore I would like her words to explain or describe my work here.
In her works Olearius addresses the calculation and significance of numerical values and their construction in the context of artistic production and presentation. In Cubicle x Factor she questions the valuation and rationalization of artworks and artistic work. Olearius presents an installation of rented artworks which values she recalculated. Her method of calculation is based on the common formula Height + Width x Factor, with the latter representing a subjective element. The key part of her installation are wrapped canvases that form a cubicle typical for office spaces – an epitome for the rationalization of office workplaces. Six of the used artworks were rented from commercial online artwork renting agency that lend artworks amongst others for the decoration of officespaces.
These rented artworks remain anonymous, therefore denying their perception as aesthetic objects. Solely their dimensions and Olearius idiosyncratic calculations which are written in fine handwriting on graph paper provide information about the underlying artworks. The artist not only recalculated their financial values but also correlates them to their respective duration of being observed. Prices are calculated for an average view time of an artwork of 11 seconds as well the desired view time of 20 minutes. Besides defining a new sale price she also calculates a new rental price and compares it to the rental fee invoiced to her by the renting agency.
On the wall next to the graph papers hangs a canvas with 6 colored stripes. The painting appears sensuous compared to the cardboard boxes and documents but is determined by rigorous calculation. Each of the 6 stripes represents the average color value of one of the paintings hidden in the cubicle. While the artists subjective calculations demonstrate the absurdity of mathematical valuation of artistic works,the painting tempts the viewer to speculate about the hidden paintings.
Nadja Quante, Artistic Director and Curator, Künstlerhaus Bremen
Hairmatic
Read more:
